Interesting things to make out of paper—from mathematical models to toys.

• Browse
• Random
• About

Weaire-Phelan Structure

Lord Kelvin posed the question of how space could be divided into cells of equal volume and minimal surface area. This is equivalent to asking what shape equal-sized soap bubbles would take in foam since bubbles naturally assume shapes with minimal surface area. He conjectured that the solution was a lattice of truncated octahedra with slightly curved hexagonal faces. The polyhedral version of this structure is called the Kelvin structure. The physicists Denis Weaire and Robert Phelan applied a structure known from crystallography to the problem and found that the so-called Weaire-Phelan structure improves on the Kelvin structure.

Steps

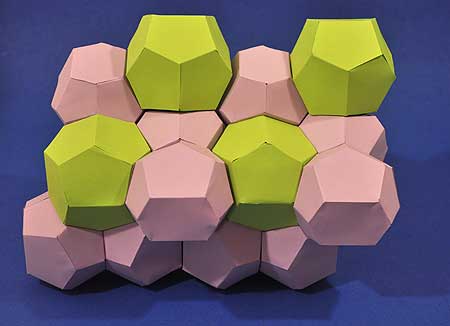
1. Cut out, score, fold and glue two copies of the dodecahedra and six copies of the 14-sided polyhedra. The dodecahedra are shown in green in the photo above.
   
2. Glue pairs of 14-sided polyhedra together at their hexagonal faces. You will have three pairs of polyhedra.
   
3. Glue a dodecahedron to one pair of 14-sided polyhedra at the top pair of pentagonal faces.
   
4. Glue a pair of 14-sided polyhedra to the left-hand side of the dodecahedron.
   
5. Glue a pair of 14-sided polyhedra to the front of the dodecahedron.
   
6. Glue a dodecahedron to the top front of the group. This completes a unit that repeats to fill space.
   

Notes

• The Beijing National Aquatics Center built for the 2008 Olympics has a design of "bubbles" enclosing the building that is based on the Weaire-Phelan structure.
  
• The Weaire-Phelan structure has not been proven to be optimal.
  

Links

• The patterns were created from a file on Thomas Girsewald's site.

References

• (Lord Kelvin 1887) (v. Girsewald)

Comments

  © Laszlo Bardos