Interesting things to make out of paper—from mathematical models to toys.
Clifford Torus
If you slice a torus (a doughnut-shaped surface) in half with a plane parallel to its axis, the cross section is two circles.
Likewise, if you cut the torus across the middle with a plane perpendicular to its axis as if you were slicing a bagel, you would see two circles: one circle going around the circumference and a smaller circle defining the hole. There is one more pair of circles hidden inside a torus that goes through any point on the torus. If you cut the torus in half at just the right slant, your cross section will be two interlocking circles called Villarceau circles. The animated image below (from Wikipedia) shows how this is possible.
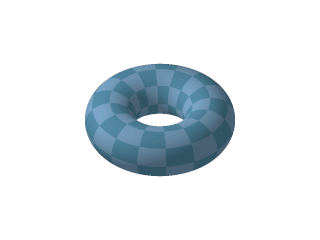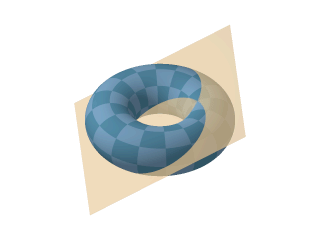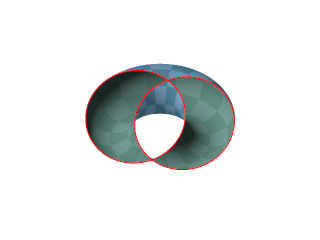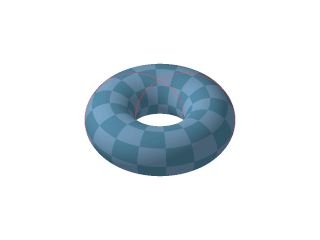
The paper model in this project shows Villarceau circles in a torus. Each seam in the model is one of the two Villarceau circles that goes through a point. The name Clifford torus refers to the fact that this torus is a stereographic projection of a 4D Clifford torus into 3D.
Video Instructions
Steps
- Cut out two copies of the pattern out of paper or card stock.
Tape the edges of neighboring curves together, placing the tape on the back of the pattern. Start taping near the middle and work your way outward. Line up the dashed lines on the pattern as you go to make sure that the edges are joined properly.
When you reach the ends of the curves, the tips should line up as shown.- Repeat these steps for the second copy of the pattern.
Tape the ends together on one of the parts. This step is tricky. If you hold the two ends as shown, you must give one of the ends a 180° twist to make them line up properly.
The part should now look like this. Here you can see the Villarceau circle clearly. You can also see the torus start to take shape. 
Tape the second part onto the first. Start at the tip and work your way around the Villarceau circle.
Links
- I learned about the Clifford torus from an article "Imaging maths - Unfolding polyhedra" by Konrad Polthier from the online Plus Magazine's Issue 27, November 2003. In the article, there is a link to a short animation of a Clifford torus folding and unfolding from its net.
- I used Mathematica code from Paul Nylander's web site to generate the coordinates that I used to make the net. I recommend visiting his site and browsing around. He discusses many interesting and beautiful mathematical topics.
