Goldberg's Tri-stable Polyhedron

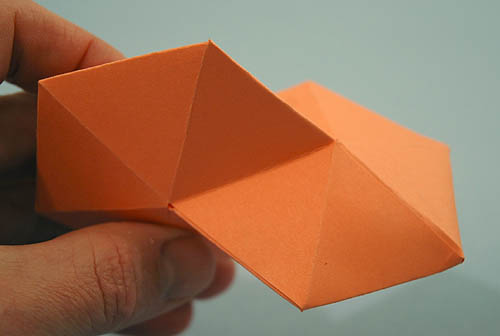
The Goldberg's tristable polyhedron is a polyhedron with three stable configurations. It looks like two pentagonal dipyramids "kissing." As one dipyramid is pinched, the other opens up. If you build the model out of card stock, you can get a sense of the movement of this polyhedron, but you will not be able to tell the three stable states. This polyhedron is sensitive to small inaccuracies. If, for example, the equilateral triangles where replaced by isosceles triangles with a vertex angle of 59 degrees (instead of 60) one pyramid can collapse flat.

Steps

1. Score the fold lines. Dashed lines are valley folds and dot-dashed liens are mountain folds.
   
2. Glue the tabs to their corresponding edges. This gets very tricky.
   

References

• (Goldberg, Unstable Polyhedral Structures 1978)